Modelling dynamics of a droplet interacting with porous surfaces
Time: Thu 2021-11-25 10.30 - 11.30
Location: FLOW eSeminar
Participating: Si Suo (University of Sydney)
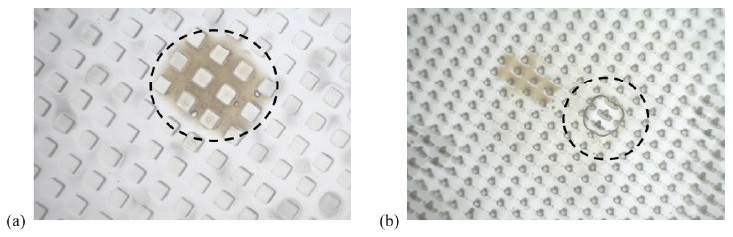
Abstract. Droplet dynamics interacting with porous surfaces, including impact, spreading, imbibition, trapping, splitting, etc., form a fundamental basis of a wide range of engineering applications, such as enhanced oil recovery and CO2 geometrical sequestration, water management in fuel cells, and surface cleaning and coating. In recent decades, various surfaces with bio-inspired microstructures are proposed to achieve certain cutting-edge functions, like anti-icing, self-cleaning, super hydrophobicity etc. However, practical application of these artificial surfaces still faces challenges, e.g., how to efficiently remove liquid stains trapped within microstructures, as shown in Figure 1, strongly determines their performance and durability. In this study, we numerically investigate the droplet behaviours within porous surfaces under shear flows. During the movement, the droplet mainly spreads along the pore space driven by the viscous shear, while a certain part of droplet would be split and attached on the obstacles as the leftover volume, owing to the capillary effects. Through a series of numerical simulations under various flow conditions, it is suggested that three droplet mobility modes can be identified, i.e., the stick-slip, crossover, and slugging mode, due to the complex competition between viscous and capillary effects. To quantitatively characterise the droplet dynamics within porous surfaces, we propose a pore-scale capillary number, particularly considering the geometrical factor, describing the non-uniformity of flow field in given structures. By comparing against the simulation results, the proposed dimensionless number presents a strong correlation with the leftover ratio. The dominating mechanisms revealed in this study provide a basis for further research on enhancing surface cleaning and optimising design of anti-fouling surfaces.